
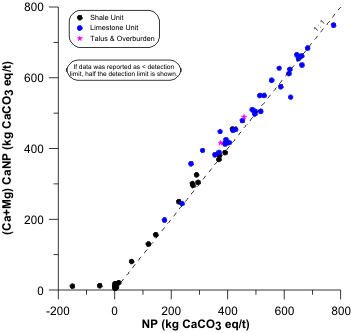
Figure 1. An Example of Limestone Rock with Measured Neutralization Potentials above 500 kg/t, Showing a 1:1 Correlation with Inorganic-Carbon-Based CaNP.
MDAG.com Internet Case Study 20
Conversion of Minerals into Neutralization Potentials
with Units of CaCO3 Equivalent
by K.A. Morin and N.M. Hutt
© 2006 Kevin A. Morin and Nora M. Hutt
Abstract
Neutralization Potential (NP) and Sulphide Acid Potentials are critical aspects for prediction of acid rock drainage (ARD), and are usually reported in units of kg CaCO3 equivalent/tonne of solid sample. Not often stated, the conversion of chemical analyses into CaCO3 equivalent involves assumptions on mineral formulas and environmental conditions, which will not apply to all minesites.
NP procedures implicitly assume that calcite (CaCO3) will neutralize only to pH below ~6.3, which probably reflects the use of excess acid in the procedures. As a result, 1000 kg of calcite/tonne will produce an NP around 1000 kg CaCO3 equivalent/tonne. In reality, ~6.3 < pH < ~10.3 is often the desirable target. In this pH range, 1000 kg of calcite/tonne will neutralize only about 500 kg CaCO3 equivalent/tonne, although its NP would be 1000 kg/t. This partly explains the generic SNPR criterion (NP/SAP) of 2.0, requiring twice as much NP than SAP.
Because silicate minerals often dissolve too slowly to be fully quantified by NP procedures, conversion factors provide an estimate of slow-releasing silicate-based NP. Again, mineral formulas and environmental conditions like pH play major roles in calculated silicate NP. For example, with the feldspar mineral Anorthite (CaAl2Si2O8(s)), at ~4.5<pH<~9.8, 1 kg anorthite/t = 0.36 kg CaCO3 equivalent. Other conversion factors can be roughly estimated by a spreadsheet that balances equations for silicate-mineral dissolution with H2O and H+.
1. Introduction
Detailed assessment and prediction of minesite-drainage chemistry require the linking of solid-phase minerals to aqueous geochemistry. Under near-surface environmental conditions, water is the medium into which minerals dissolve, and water is even a necessary reactant for some minerals. This is the reason dry minerals do not have a pH, but “paste” and “rinse” pH can be measured when water is added to the minerals.
For acidic rock drainage (ARD), one well-known conversion factor is 31.25. This converts a solid-phase sulphide level, as %S, into aqueous acidity, in units of kg CaCO3 equivalent/tonne of solid sample. These units are the same as t CaCO3 equivalent/1000 t and parts-per-thousand CaCO3 equivalent. The result is labelled, Sulphide Acid Potential (SAP).
An opposing Neutralization Potential (NP) is also determined in the same units as SAP. A Sulphide Net Potential Ratio (SNPR = NP / SAP) can then be used to predict whether ARD might ever be released from the solid sample. SAP, NP, and SNPR are all part of acid-base accounting (ABA).
This document focusses on the meaning of CaCO3 equivalent, and its implications for SAP and NP.
2. Conversion of Solid-Phase Sulphur to CaCO3 equivalent
Morin (1990) explained that a value of 31.25 assumed a great deal about the local environmental conditions, which may not be appropriate for sulphidic rock at all minesites. These conditions are:
1) solid-phase sulphur occurs only as S22-;
2) S22- oxidizes completely to sulphate;
3) pyrite (FeS2) is the only sulphide mineral;
4) molecular oxygen and water are the only oxidants;
5) all iron oxidizes to the ferric (Fe3+) state; and,
6) all iron precipitates from water as Fe(OH)3 which generally implies aqueous pH > ~3.5.
This is reflected in the “standard” pyrite-oxidation equation:
FeS2 + 7/2 H2O + 15/4 O2 → Fe(OH)3 + 2 SO42- + 4H+ (Eq. 1)
If all six conditions are not applicable, Equation 1 would not apply and the conversion factor for sulphur could be substantially different. Morin (1990) provided some examples where conversion factors could range from zero to 125.0.
3. A Closer Look at “CaCO3 equivalent”
There is another important aspect to the value of 31.25. It is not an aqueous condition, but a human construct that greatly complicates the concepts of NP and SAP and their comparison. This is the definition of one mole of aqueous acidity, as “CaCO3 equivalent”, as equal to two moles of aqueous H+.
1 mol CaCO3 equivalent = 2 mol aqueous H+ (Eq. 2)
Equation 2 applies to acidity in water, but the units contain “CaCO3” which is a solid mineral. This construct can be traced to standard methods for analyses of aqueous acidity and alkalinity.
Without water, an acid-generating mineral cannot generate aqueous acidity, and an acid-neutralizing mineral cannot generate aqueous alkalinity. A source of confusion lies in the definitions of acidity and alkalinity. These two parameters are not “real” in the sense of a chemical element, but often represent non-linear combinations of elements and aqueous species, defined by titrations to arbitrary endpoint pH values. Kirby and Cravotta (2005a and 2005b) highlighted the variability and confusion over various definitions of acidities and alkalinities. This would be enough to render acidity, defined in our case by “CaCO3 equivalent”, ambiguous or inappropriate in some cases. Nevertheless, we will continue using Equation 2 as our simple definition, to explore further the connection of CaCO3 equivalent to minerals.
4. Conversion of Solid-Phase CaCO3 to CaCO3 Equivalent
So what is the relationship of CaCO3 equivalent to mineralogy? The basic answer is: the relationship is highly site-specific, like the six environmental conditions in Equation 1, and thus the relationship is variable and sometimes confusing.
Simplistically, calcite, a solid mineral with the formula CaCO3, dissolves into water to neutralize acidity. Thus, there is a relationship of CaCO3 (the solid mineral) and CaCO3 equivalent (the amount of aqueous acidity neutralized by dissolution of calcite), but the ratio is not necessarily 1:1. The ratio actually depends on environmental conditions, like aqueous pH.
When pH remains below ~6.3, one mole of CaCO3 (calcite) dissolves to neutralize two moles of acidity, which is one mole of CaCO3 equivalent:
pH <~6.3: CaCO3 + 2H+ ↔ Ca2+ + H2CO30 ↔ Ca2+ + H2O + CO2(gas) (Eq. 3a)
Because solid calcite and aqueous CaCO3 equivalent have the same molecular weight, 1 kg/t of calcite neutralizes 1 kg CaCO3 equivalent/t in the water surrounding the calcite.
pH<~6.3: 1 kg CaCO3(solid)/t = 1 kg CaCO3 equivalent(aqueous)/t (Eq. 3b)
However, between pH ~6.3 and ~10.3, 2 kg/t of calcite are required to neutralize 1 kg CaCO3 equivalent/t:
~6.3<pH<~10.3: 2CaCO3 + 2H+ ↔ Ca2+ + 2HCO3- (Eq. 4a)
~6.3<pH<~10.3: 1 kg CaCO3(solid)/t = 0.5 kg CaCO3 equivalent (aqueous)/t (Eq. 4b)
Again, there are other conditions affecting the ratio, but pH and mineralogy are the focus here.
Because nearly complete neutralization above pH 6 is often the target with minesite-drainage chemistry, 1 weight-percent (wt-%) calcite based on mineralogy of a sample will provide only 0.5 wt-% (or 5 kg/t) CaCO3 equivalent/t of neutralization (Equation 4b). Even this scenario is simplified, so site-specific variations in the ratio can be expected.
Theoretically, mostly pure carbonate rock like limestone should not have an NP greater than roughly 500 kg/t based on Equation 4. However, this is often not the case (e.g., Figure 1) and the standard expectation is “the NP of calcite is 1000 [CaCO3 equivalent]” (e.g., Jambor et al., 2006). Also, where carbonate comprises most of the NP, a good correlation of carbonate (mathematically converted to CaCO3 equivalent) to NP would be 2:1. The same would apply to (Ca+Mg) converted to (Ca+Mg)CaNP in units of CaCO3 equivalent. However, this is not often the case and a 1:1 correlation is seen instead (Figures 1 and 2). Therefore, NP implicitly assumes pH remains below ~6.3 (Equation 3), which is consistent with the Sobek and Modified NP evaluating the dissolution of neutralizing minerals in excess acid. Above ~pH 6.3, the calcite becomes less effective, explaining in part the generic ABA screening NPR (NP/SAP) criterion of 2.0.

The next issue is converting non-carbonate minerals into CaCO3 equivalent/t.
5. Conversion of Other Minerals to CaCO3 Equivalent
As explained above, 1 kg/t of solid-phase CaCO3 does not necessarily provide aqueous neutralization of 1 kg/t of CaCO3 equivalent. Therefore, when calculating the neutralization from other minerals, it is important to distinguish between the conversions for (1) an equivalent amount of solid-phase CaCO3 and (2) the aqueous neutralization in CaCO3 equivalent.
Morin and Hutt (1994) discussed the mathematical conversion of silicate minerals to solid-phase calcite and to Neutralization Potential (NP) in CaCO3 equivalent. Although the neutralization equations were corrected, the conversion factors in their Table 2 were incorrect and later revised by Morin and Hutt (1997 and 2001). Still, the factors involved several restrictive assumptions like those above for Equation 1.
5.1 Anorthite
For anorthite (calcium plagioclase, An100), the neutralization reaction, above a sufficiently high pH (~4.5) so that all aluminum precipitates as Al(OH)3, and below a pH (~9.8) where all silicon occurs as silicic acid, is:
~4.5<pH<~9.8: CaAl2Si2O8(s) + 2H+ + 6H2O → Ca2+ + 2Al(OH)3(s) + 2H4SiO40 (Eq. 5a)
This means that one mole of anorthite neutralizes two moles of acidity, which is one mole of CaCO3 equivalent. Because the molecular weight of anorthite is 278,
~4.5<pH<~9.8: 1 kg anorthite/t = 0.36 kg CaCO3 equivalent (Eq. 5b)
At pH below ~4.5, Al(OH)3 will not precipitate and thus anorthite would neutralize more acidity than Equation 5b. At pH above ~9.8, silicic acid will dissociate, releasing H+, and thus anorthite would neutralize less acidity than Equation 5b.
The conversion of the neutralizing capacity of anorthite to the neutralizing capacity of solid-phase calcite depends on the pH (Equations 3 and 4), and thus one mole of anorthite may be equivalent to one mole or one-half mole of calcite, depending on pH. Thus,
~4.5<pH<~6.3: 1 kg anorthite/t = 0.36 kg solid-phase calcite/t (Eq. 6a)
~6.3<pH<~9.8: 1 kg anorthite/t = 0.72 kg solid-phase calcite/t (Eq. 6b)
Because we are usually interested in Neutralization Potential (NP) and neutralization of aqueous acidity, Equations 5 and 6a are often more important than Equation 6b.
5.2 Other Silicate Minerals
Plagioclase represents a series of feldspar minerals, ranging from calcium-based anorthite (CaAl2Si2O8) to sodium-based albite (NaAlSi3O8). Other minerals, like biotite, have a range of potential compositions that vary from site to site. Thus, conversion factors for these minerals vary with (1) site-specific elemental composition and (2) local environmental conditions such as pH. This greatly complicates the evaluation of the factors beyond the simple approach used here.
To simplify the calculations, we use a simple spreadsheet-based calculator, called “NP Stoichiometry”. This spreadsheet accepts as input the stoichiometry of Ca, Mg, Na, K, Fe(III), Al, Si, O, and H within a silicate mineral (e.g., anorthite is 1 Ca, 2Al, 2 Si, 8O). The spreadsheet then calculates the amount of H2O needed to balance O (Equation 5a). Then, the remaining number of H+ needed to balance the reaction is assigned to acid generation (if the H+ value is negative) or to acid neutralization (if the value is positive). Assumed conditions include all Fe3+ and Al are precipitated as hydroxides and silicic acid is the only aqueous form of silicon, which is consistent with relatively aerobic, near-neutral conditions. For example, various forms of hornblende can neutralize roughly 4 to 6 moles of H+, which is 2 to 3 moles of CaCO3 equivalent.
6. Conclusion
Conversion factors of solid-phase minerals to aqueous acidity or neutralization, as CaCO3 equivalent, depend on mineral formulas and environmental conditions like pH. As a result, no simple table of conversion factors is reliable in all situations, but more complex calculations may be needed. These can be accomplished with a mass-balance spreadsheet, such as “NP Stoichiometry”.
References
Jambor, J.L., J.E. Dutrizac, and M. Raudsepp. 2006. Comparison of measured and mineralogically predicted values of the Sobek Neutralization Potential for intrusive rocks. IN: R.I. Barnhisel, ed., Proceedings of the 7th International Conference on Acid Rock Drainage (ICARD), p.820-832, March 26-30, 2006, St. Louis, MO, USA.
Kirby, C,S., and C.A. Cravotta III. 2005a. Net alkalinity and net acidity 1: Theoretical considerations. Applied Geochemistry, 20, p. 1920-1940.
Kirby, C,S., and C.A. Cravotta III. 2005b. Net alkalinity and net acidity 2: Practical considerations. Applied Geochemistry, 20, p. 1941-1964.
Morin, K.A. 1990. Problems and proposed solutions in predicting acid drainage with acid-base accounting. IN: Acid Mine Drainage - Designing for Closure, Geological Association of Canada/Mineralogical Association of Canada Conference, Vancouver, British Columbia, May 16-18, p.93-107, p. 93-107.
Morin, K.A., and N.M. Hutt. 2001. Environmental Geochemistry of Minesite Drainage: Practical Theory and Case Studies - Digital Edition. MDAG Publishing (www.mdag.com), Surrey, Canada. ISBN 0-9682039-1-4.
Morin, K.A., and N.M. Hutt. 1997. Environmental Geochemistry of Minesite Drainage: Practical Theory and Case Studies. MDAG Publishing (www.mdag.com), Surrey, Canada. ISBN 0-9682039-0-6.
Morin, K.A., and N.M. Hutt. 1994. Observed preferential depletion of neutralization potential over sulfide minerals in kinetic tests: Site-specific criteria for safe NP/AP ratios. IN: Proceedings of the Third International Conference on the Abatement of Acidic Drainage, Pittsburgh, Pennsylvania, USA, April 24-29, Volume 1, p.148-156.
© 2006 Kevin A. Morin and Nora M. Hutt
For more case studies, see Environmental Geochemistry of Minesite
Drainage: Practical Theory and Case Studies.
Created by K.Morin